Triple Hexiamonds

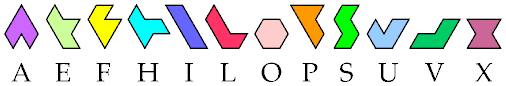
This page shows figures that can be tiled by each of three hexiamonds. It was inspired by Livio Zucca's Triple Pentominoes. For figures that can be tiled by larger groups of hexiamonds, see Multiple Compatibility for Polyiamonds.

Where no closed solution is known, a reëntrant solution is given. If you find a smaller solution, or a closed solution where none is given, please write.

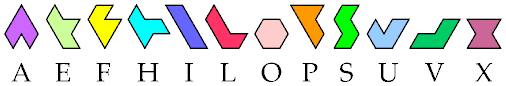
A-E-F : ∞	A-E-H : 6	A-E-I : ∞	A-E-L : 3	A-E-O : ∞
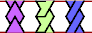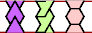
A-E-P : 72	A-E-S : ∞	A-E-U : 3	A-E-V : ∞	A-E-X : ∞
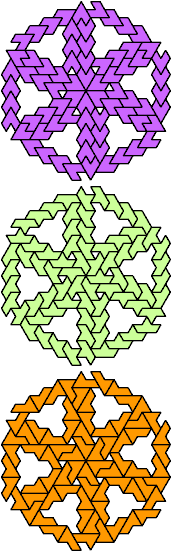A-F-H : 12	A-F-I : 2	A-F-L : 6	A-F-O : ∞	A-F-P : 2
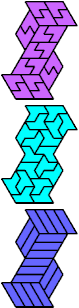
A-F-S : 54	A-F-U : 6	A-F-V : 2	A-F-X : ∞	A-H-I : 18
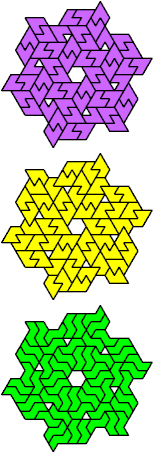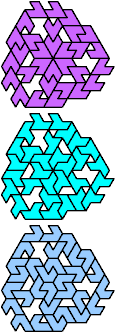
A-H-L : 9	A-H-O : ∞	A-H-P : 12	A-H-S : 54	A-H-U : 30
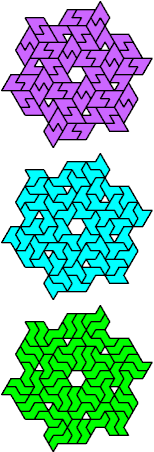A-H-V : 18	A-H-X : ∞	A-I-L : 6	A-I-O : ∞	A-I-P : 2
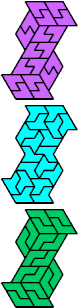A-I-S : 126	A-I-U : 18	A-I-V : 2	A-I-X : ∞	A-L-O : ∞
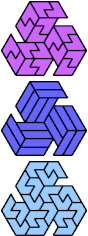A-L-P : 6	A-L-S : 54	A-L-U : 3	A-L-V : 6	A-L-X : ∞
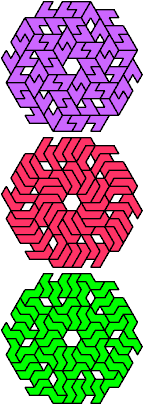
A-O-P : ∞	A-O-S : ∞	A-O-U : ∞	A-O-V : ∞	A-O-X : ∞
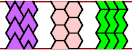A-P-S : ∞	A-P-U : 18	A-P-V : 2	A-P-X : ∞	A-S-U : ∞
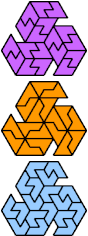A-S-V : ∞	A-S-X : ∞	A-U-V : 18	A-U-X : ∞	A-V-X : ∞
E-F-H : 30	E-F-I : ∞	E-F-L : 6	E-F-O : ∞	E-F-P : 6
E-F-S : ∞	E-F-U : 6	E-F-V : ∞	E-F-X : ∞	E-H-I : 3
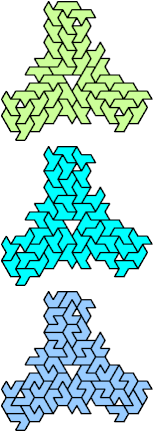
E-H-L : 3	E-H-O : ∞	E-H-P : 6	E-H-S : 6	E-H-U : 42
E-H-V : 6	E-H-X : 3	E-I-L : 3	E-I-O : ∞	E-I-P : 6
E-I-S : ∞	E-I-U : ∞	E-I-V : 6	E-I-X : ∞	E-L-O : ∞
E-L-P : 6	E-L-S : 18	E-L-U : 3	E-L-V : ∞	E-L-X : 3
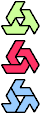E-O-P : ∞	E-O-S : ∞	E-O-U : ∞	E-O-V : ∞	E-O-X : ∞
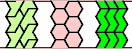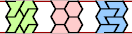
E-P-S : 6	E-P-U : 6	E-P-V : 6	E-P-X : 6	E-S-U : ∞
E-S-V : ∞	E-S-X : ∞	E-U-V : ∞	E-U-X : ∞	E-V-X : ∞
F-H-I : 6	F-H-L : 6	F-H-O : 6	F-H-P : 2	F-H-S : 2
F-H-U : 2	F-H-V : 6	F-H-X : ∞	F-I-L : 6	F-I-O : ∞
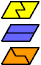
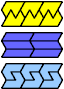
F-I-P : 2	F-I-S : 6	F-I-U : 6	F-I-V : 2	F-I-X : ∞
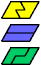
F-L-O : 6	F-L-P : 6	F-L-S : 6	F-L-U : 2	F-L-V : 6
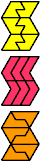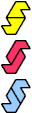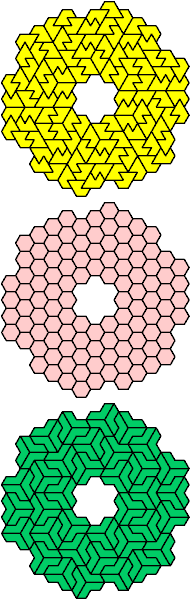
F-L-X : ∞	F-O-P : 6	F-O-S : 108	F-O-U : 42	F-O-V : 108
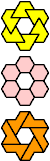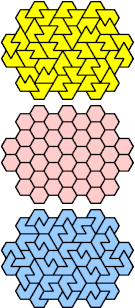F-O-X : ∞	F-P-S : 2	F-P-U : 2	F-P-V : 2	F-P-X : 6
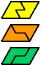F-S-U : 2	F-S-V : 66	F-S-X : ∞	F-U-V : 6	F-U-X : ∞
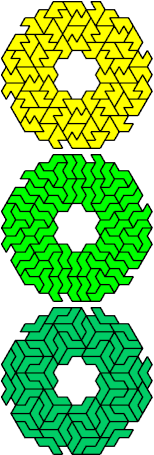F-V-X : ∞	H-I-L : 3	H-I-O : ∞	H-I-P : 6	H-I-S : 6
H-I-U : 6	H-I-V : 6	H-I-X : ∞	H-L-O : 6	H-L-P : 2
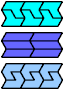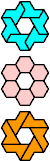
H-L-S : 6	H-L-U : 6	H-L-V : 9	H-L-X : 3	H-O-P : 6
H-O-S : 18	H-O-U : 18	H-O-V : 18	H-O-X : ∞	H-P-S : 2
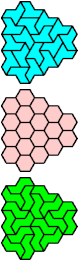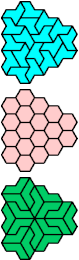H-P-U : 2	H-P-V : 6	H-P-X : 6	H-S-U : 2	H-S-V : 3
H-S-X : ∞	H-U-V : 6	H-U-X : ∞	H-V-X : ∞	I-L-O : ∞
I-L-P : 6	I-L-S : 9	I-L-U : 3	I-L-V : 6	I-L-X : ∞
I-O-P : ∞	I-O-S : ∞	I-O-U : ∞	I-O-V : ∞	I-O-X : ∞
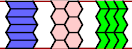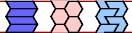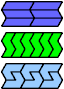
I-P-S : 6	I-P-U : 6	I-P-V : 2	I-P-X : ∞	I-S-U : 6
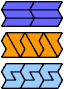I-S-V : 27	I-S-X : ∞	I-U-V : 18	I-U-X : ∞	I-V-X : ∞
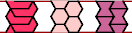
L-O-P : 6	L-O-S : 18	L-O-U : 15	L-O-V : 18	L-O-X : ∞
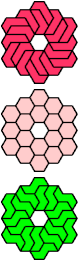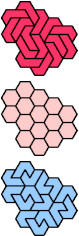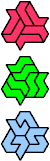
L-P-S : 6	L-P-U : 6	L-P-V : 6	L-P-X : ∞	L-S-U : 6
L-S-V : 9	L-S-X : ∞	L-U-V : 18	L-U-X : ∞	L-V-X : ∞
O-P-S : 6	O-P-U : 6	O-P-V : 6	O-P-X : ∞	O-S-U : 3
O-S-V : 3	O-S-X : ∞	O-U-V : 3	O-U-X : ∞	O-V-X : ∞
P-S-U : 2	P-S-V : 6	P-S-X : ∞	P-U-V : 6	P-U-X : ∞
P-V-X : ∞	S-U-V : 3	S-U-X : ∞	S-V-X : ∞	U-V-X : ∞

Last revised 2014-02-18.