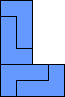

This tiling is said to be rep-4 (or rep-22), because it uses four tiles.
Polycubes are polyforms made by joining equal cubes face to face. Little is known about polycube rep-tilings, because computing polycube tilings is lengthy and complex.
Any polycube that can tile a rectangular box is a reptile. Rectangular boxes can always be joined to form a cube, and copies of this cube can form an enlarged copy of the original polycube. For example, the U pentacube can form boxes with dimensions 2×3×5 and 4×4×5, and such boxes can tile a cube of side 10. So the U pentacube has a rep-1000 tiling. I show below that it also has a rep-27 tiling.
The smallest polycubes that cannot reptile themselves (nor tile any box) are the G and X pentacubes:
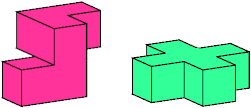
For the smallest box tilings of the other pentacubes, see Pentacubes in a Box. On this page I show only the smallest known rep-tiling for each polycube. If the rep-tiling is assembled from big cubes, I give only a description of the tiling. It would be interesting to know all possible rep-tiling values for each polycube.
Polycubes of orders 4 and up may be chiral, which means that they have distinct mirror images. Some chiral polyforms have known rep-tilings only if reflection is allowed. If a polyform can tile an enlarged copy of its mirror image without reflection, it can also tile an enlarged copy of itself without reflection, by iterating the mirror-image tiling.
If you find a small solution for a case not shown here, please write!
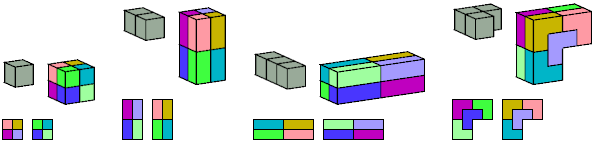
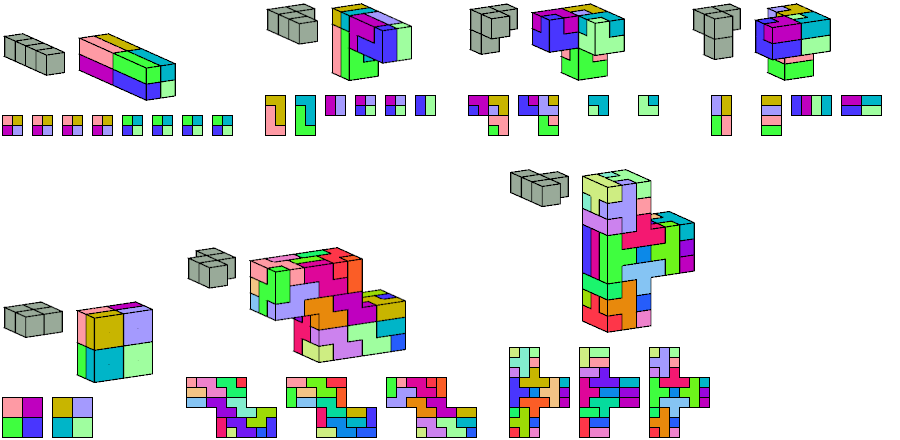
Mike Reid first solved pentacube U at scale 3 and pentacube K at scale 4. Andrew Clarke first solved pentacubes Q and Y at scale 4.
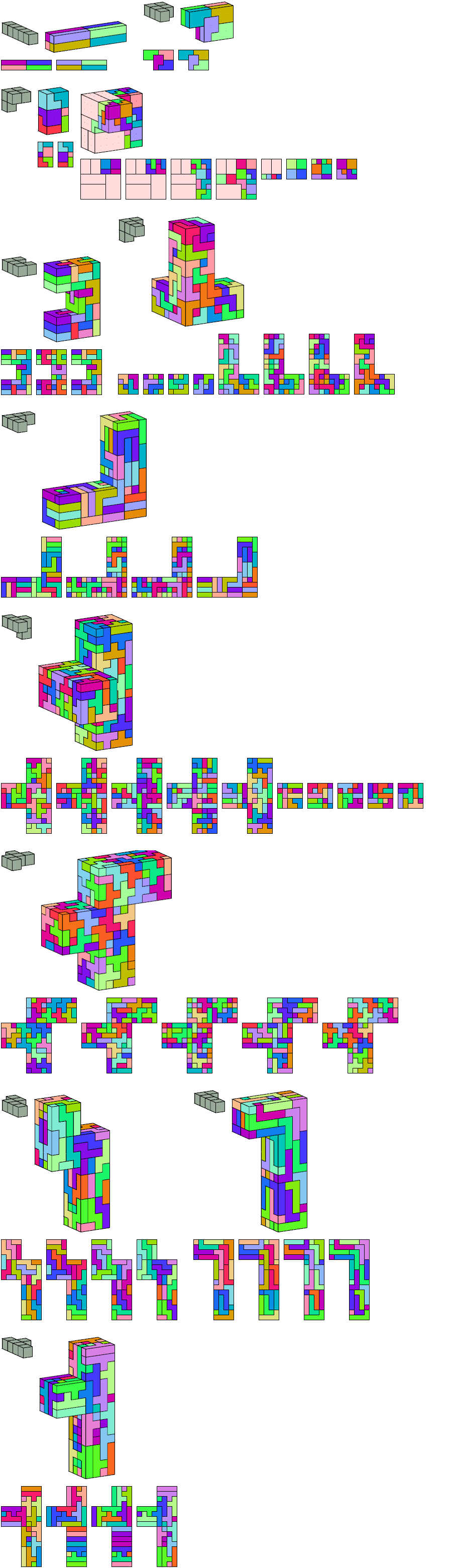
| Pentacube | Boxes | Cube | Tiles | Minimal? | |
|---|---|---|---|---|---|
| A |  | 6×10×10
8×10×10 | 20×20×20 | 8000 | — |
| M |  | 10×10×20 | 20×20×20 | 8000 | — |
| T |  | 10×10×10 | 10×10×10 | 1000 | — |
| W |  | 5×10×10 | 10×10×10 | 1000 | — |
| Z |  | 10×10×10 | 10×10×10 | 1000 | — |

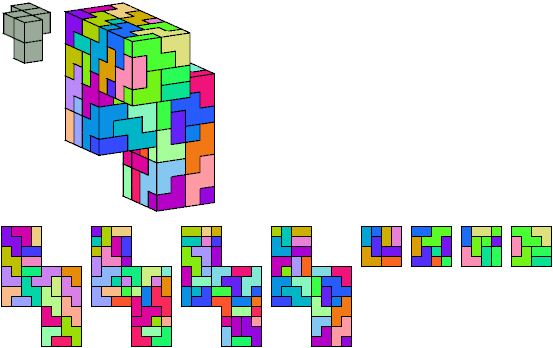
| Pentacube | Boxes | Cube | Tiles | Minimal? | |
|---|---|---|---|---|---|
| R |  | 5×10×10 | 10×10×10 | 1000 | — |
| S |  | 4×10×10
6×10×10 | 10×10×10 | 1000 | — |
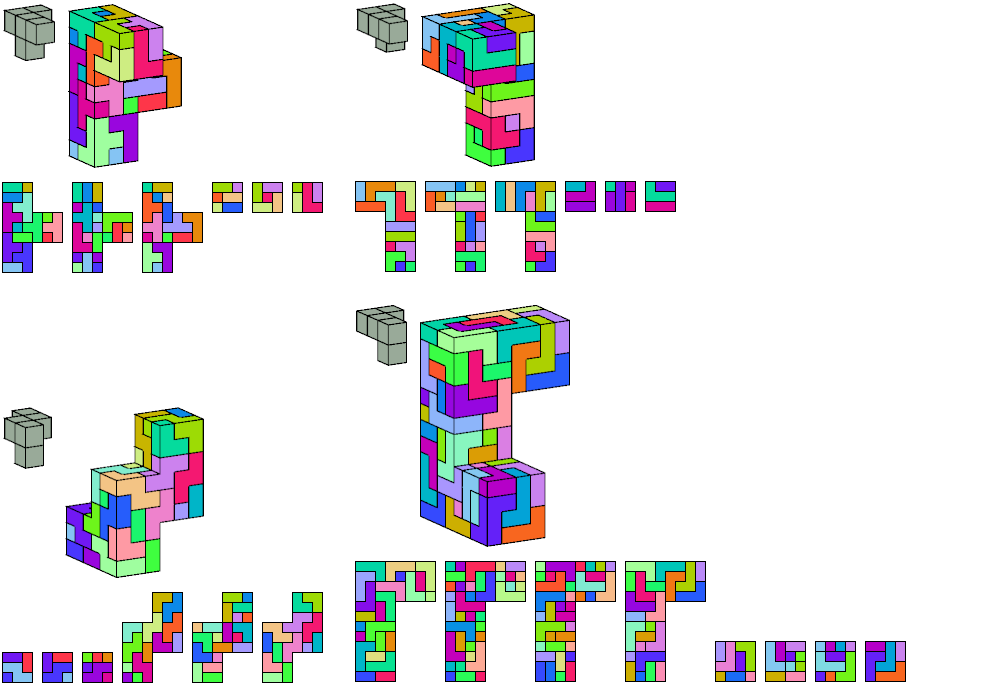
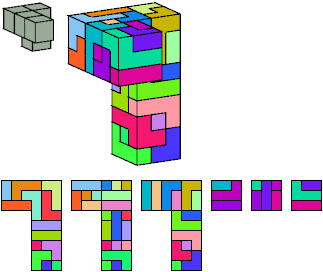
| Pentacube | Boxes | Cube | Tiles | Minimal? | |
|---|---|---|---|---|---|
| R |  | 2×10×10 | 10×10×10 | 1000 | — |
Last revised 2018-09-01.