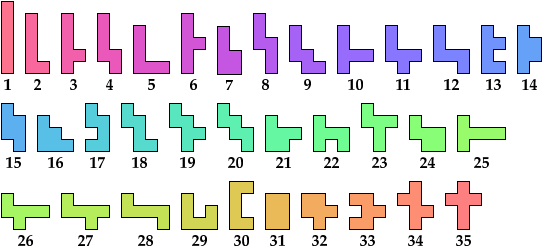
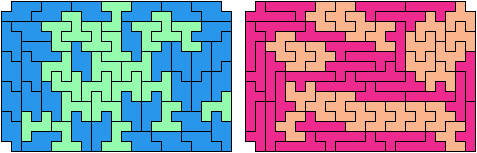
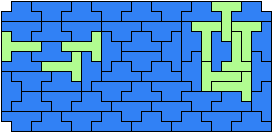
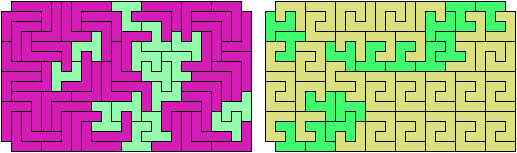
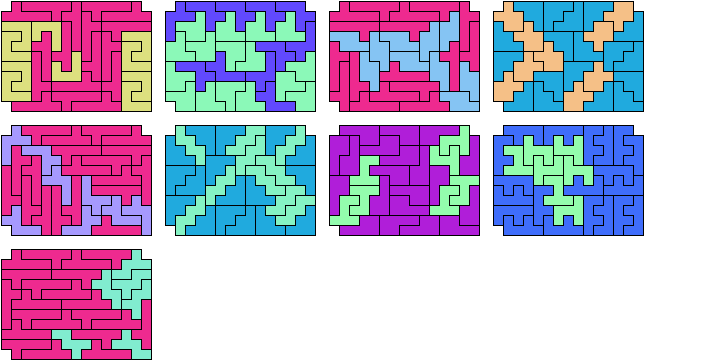
The problem of arranging copies of a polyomino to form a rectangle has been studied for a long time. Here I study the problem of arranging copies of two hexominoes to form a rectangle with three corner cells removed.
If you find a smaller solution or solve an unsolved case, please write.
See also

| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34 | 35 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | * | × | 7 | × | × | × | × | × | × | × | ? | × | 16 | × | ? | 16 | × | × | ? | × | × | 25 | × | × | ? | × | ? | × | × | × | × | 4 | × | ? | × |
| 2 | × | * | 3 | × | × | × | × | × | × | × | 4 | × | 10 | × | 10 | 10 | × | × | 19 | × | × | 17 | × | × | 10 | × | 10 | × | × | × | × | 13 | × | ? | × |
| 3 | 7 | 3 | * | 31 | 10 | ? | 10 | 45 | 17 | ? | 27 | 32 | 17 | 19 | 27 | 17 | 19 | 45 | 27 | 37 | 16 | 47 | 42 | 16 | 45 | 25 | ? | ? | 27 | ? | 16 | 47 | 57 | ? | ? |
| 4 | × | × | 31 | * | × | × | × | × | × | × | ? | × | 16 | × | 2 | 19 | × | × | ? | × | × | 19 | × | × | 37 | × | ? | × | × | × | × | ? | × | ? | × |
| 5 | × | × | 10 | × | * | × | × | × | × | × | ? | × | 13 | × | ? | 16 | × | × | 42 | × | × | 62 | × | × | ? | × | 37 | × | × | × | × | 37 | × | ? | × |
| 6 | × | × | ? | × | × | * | × | × | × | × | ? | × | 42 | × | ? | ? | × | × | 47 | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 7 | × | × | 10 | × | × | × | * | × | × | × | 16 | × | 22 | × | 2 | 13 | × | × | 16 | × | × | 27 | × | × | 16 | × | 19 | × | × | × | × | 19 | × | ? | × |
| 8 | × | × | 45 | × | × | × | × | * | × | × | ? | × | ? | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 9 | × | × | 17 | × | × | × | × | × | * | × | ? | × | 17 | × | 2 | 24 | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 10 | × | × | ? | × | × | × | × | × | × | * | ? | × | 32 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | 71 | × | ? | × |
| 11 | ? | 4 | 27 | ? | ? | ? | 16 | ? | ? | ? | * | ? | ? | 32 | ? | ? | ? | ? | ? | ? | 27 | 47 | ? | 2 | ? | ? | ? | ? | 38 | ? | 2 | ? | ? | ? | ? |
| 12 | × | × | 32 | × | × | × | × | × | × | × | ? | * | 32 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 13 | 16 | 10 | 17 | 16 | 13 | 42 | 22 | ? | 17 | 32 | ? | 32 | * | 16 | 17 | ? | 2 | 45 | 45 | 76 | 22 | 27 | 71 | 45 | ? | 37 | 45 | ? | ? | 45 | 49 | 3 | 17 | ? | ? |
| 14 | × | × | 19 | × | × | × | × | × | × | × | 32 | × | 16 | * | 32 | 13 | × | × | 32 | × | × | 45 | × | × | 58 | × | 52 | × | × | × | × | 17 | × | ? | × |
| 15 | ? | 10 | 27 | 2 | ? | ? | 2 | ? | 2 | ? | ? | ? | 17 | 32 | * | 47 | ? | ? | ? | ? | 22 | 57 | ? | 7 | ? | ? | ? | ? | 37 | ? | ? | ? | ? | ? | ? |
| 16 | 16 | 10 | 17 | 19 | 16 | ? | 13 | ? | 24 | ? | ? | ? | ? | 13 | 47 | * | ? | ? | ? | 27 | 24 | ? | ? | 13 | ? | ? | ? | ? | ? | ? | ? | 27 | ? | ? | ? |
| 17 | × | × | 19 | × | × | × | × | × | × | × | ? | × | 2 | × | ? | ? | * | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | 42 | × | ? | × |
| 18 | × | × | 45 | × | × | × | × | × | × | × | ? | × | 45 | × | ? | ? | × | * | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 19 | ? | 19 | 27 | ? | 42 | 47 | 16 | ? | ? | ? | ? | ? | 45 | 32 | ? | ? | ? | ? | * | ? | 37 | ? | ? | ? | ? | ? | ? | ? | 72 | ? | ? | ? | ? | ? | ? |
| 20 | × | × | 37 | × | × | × | × | × | × | × | ? | × | 76 | × | ? | 27 | × | × | ? | * | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 21 | × | × | 16 | × | × | × | × | × | × | × | 27 | × | 22 | × | 22 | 24 | × | × | 37 | × | * | 37 | × | × | 37 | × | 37 | × | × | × | × | 2 | × | ? | × |
| 22 | 25 | 17 | 47 | 19 | 62 | ? | 27 | ? | ? | ? | 47 | ? | 27 | 45 | 57 | ? | ? | ? | ? | ? | 37 | * | ? | ? | ? | ? | ? | ? | 62 | ? | ? | 3 | ? | ? | ? |
| 23 | × | × | 42 | × | × | × | × | × | × | × | ? | × | 71 | × | ? | ? | × | × | ? | × | × | ? | * | × | ? | × | ? | × | × | × | × | ? | × | ? | × |
| 24 | × | × | 16 | × | × | × | × | × | × | × | 2 | × | 45 | × | 7 | 13 | × | × | ? | × | × | ? | × | * | 37 | × | ? | × | × | × | × | 2 | × | ? | × |
| 25 | ? | 10 | 45 | 37 | ? | ? | 16 | ? | ? | ? | ? | ? | ? | 58 | ? | ? | ? | ? | ? | ? | 37 | ? | ? | 37 | * | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? |
| 26 | × | × | 25 | × | × | × | × | × | × | × | ? | × | 37 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | * | ? | × | × | × | × | ? | × | ? | × |
| 27 | ? | 10 | ? | ? | 37 | ? | 19 | ? | ? | ? | ? | ? | 45 | 52 | ? | ? | ? | ? | ? | ? | 37 | ? | ? | ? | ? | ? | * | ? | ? | ? | 32 | ? | ? | ? | ? |
| 28 | × | × | ? | × | × | × | × | × | × | × | ? | × | ? | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | * | × | × | × | ? | × | ? | × |
| 29 | × | × | 27 | × | × | × | × | × | × | × | 38 | × | ? | × | 37 | ? | × | × | 72 | × | × | 62 | × | × | ? | × | ? | × | * | × | × | ? | × | ? | × |
| 30 | × | × | ? | × | × | × | × | × | × | × | ? | × | 45 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | * | × | ? | × | ? | × |
| 31 | × | × | 16 | × | × | × | × | × | × | × | 2 | × | 49 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | 32 | × | × | × | * | ? | × | ? | × |
| 32 | 4 | 13 | 47 | ? | 37 | ? | 19 | ? | ? | 71 | ? | ? | 3 | 17 | ? | 27 | 42 | ? | ? | ? | 2 | 3 | ? | 2 | ? | ? | ? | ? | ? | ? | ? | * | 2 | ? | ? |
| 33 | × | × | 57 | × | × | × | × | × | × | × | ? | × | 17 | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | 2 | * | ? | × |
| 34 | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | ? | * | ? |
| 35 | × | × | ? | × | × | × | × | × | × | × | ? | × | ? | × | ? | ? | × | × | ? | × | × | ? | × | × | ? | × | ? | × | × | × | × | ? | × | ? | * |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34 | 35 |

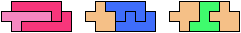
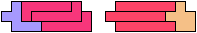
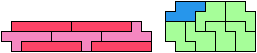
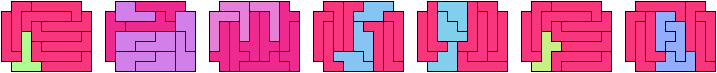
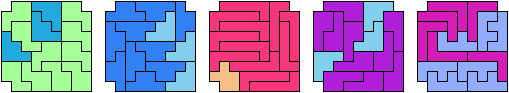
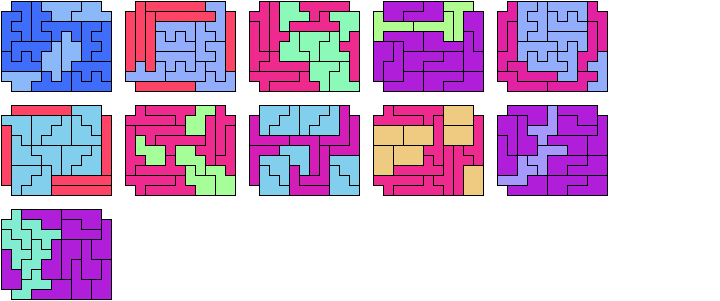
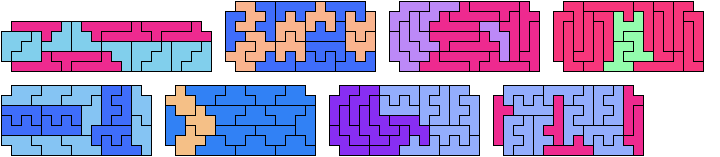
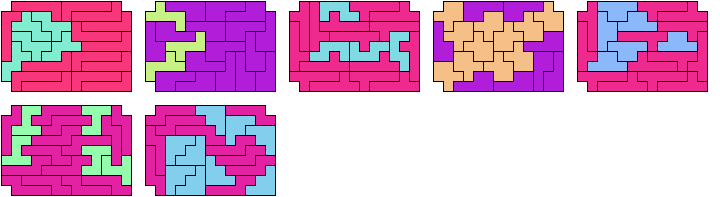
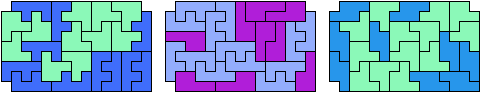
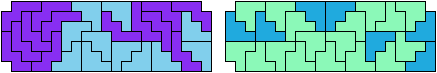
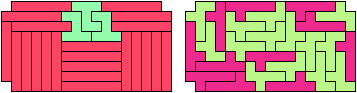
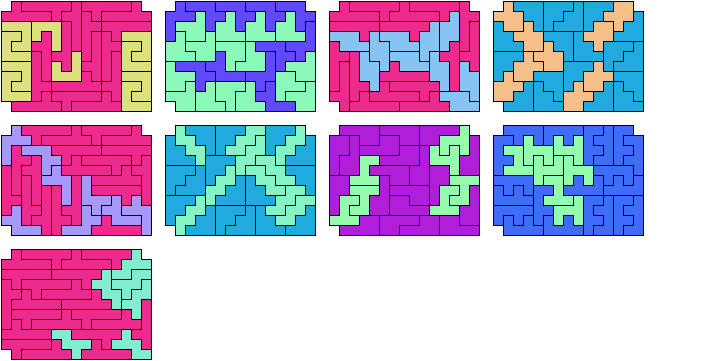

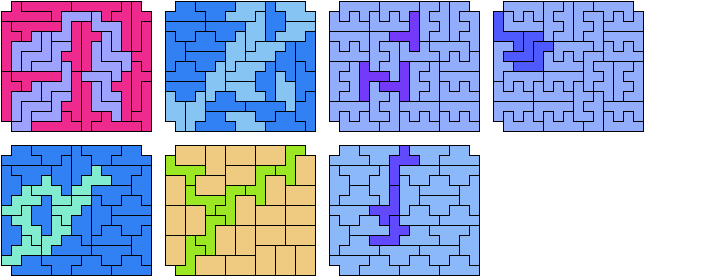
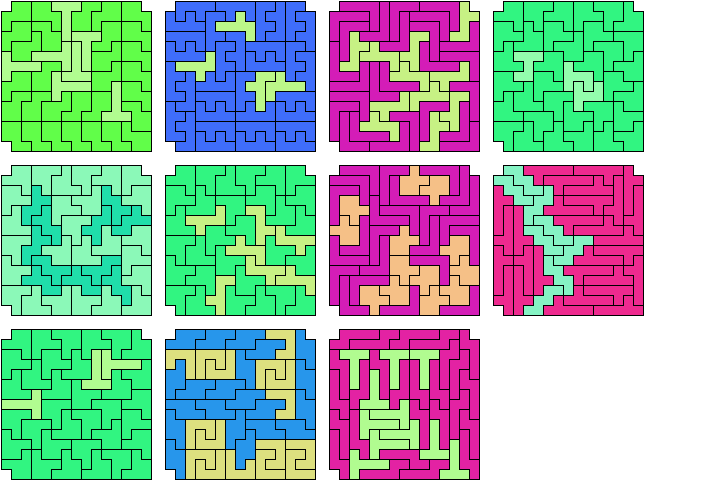
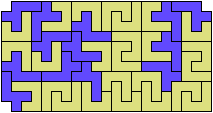


Last revised 2023-06-19.